Radial breathing-mode frequency of elastically confined spherical nanoparticles subjected to circumferential magnetic field |
| |
| Affiliation: | 1. School of Mechanical Engineering, Shiraz University, Shiraz 71963-16548, Islamic Republic of Iran;2. School of Engineering, University of the West of Scotland, Scotland;3. College of Engineering, Swansea University, UK;1. Donbass State Engineering Academy, Kramatorsk 84313, Ukraine;2. Grupo de Materia Condensada-UdeA, Instituto de Física, Facultad de Ciencias Exactas y Naturales, Universidad de Antioquia-UdeA, Calle 70 No. 52-21, Medellín, Colombia;3. Escuela de Ingeniería de Antioquia-EIA, Medellín, Colombia;4. Facultad de Ciencias, Universidad Autónoma del Estado de Morelos, Ave. Universidad 1001, CP 62209 Cuernavaca, Morelos, México;5. Institute of Physics, Kyiv 80028, Ukraine;1. Institute of Strength Physics and Materials Science of SB RAS, 2/4 Akademichesky Avenue, Tomsk 634021, Russia;2. V.D. Kuznetsov Siberian Physical Technical Institute of Tomsk State University, 1 Novosobornaja Square, Tomsk 634050, Russia;1. Instituto de Física, Universidade Federal de Mato Grosso, 78060-900 Cuiabá, Mato Grosso, Brazil;2. Departamento de Física, Universidade Federal do Ceará, Caixa Postal 6030, 60455-900 Fortaleza, CE, Brazil;3. Faculdade de Ciências Aplicadas, Universidade Estadual de Campinas - UNICAMP, R. Pedro Zaccaria, 1300, 13484-350 Limeira, SP, Brazil |
| |
| Abstract: | 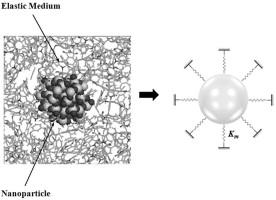
Knowledge of the vibrational properties of nanoparticles is of fundamental interest since it is a signature of their morphology, and it can be utilized to characterize their physical properties. In addition, the vibration characteristics of the nanoparticles coupled with surrounding media and subjected to magnetic field are of recent interest. This paper develops an analytical approach to study the radial breathing-mode frequency of elastically confined spherical nanoparticles subjected to magnetic field. Based on Maxwell's equations, the nonlocal differential equation of radial motion is derived in terms of radial displacement and Lorentz's force. Bessel functions are used to obtain a frequency equation. The model is justified by a good agreement between the results given by the present model and available experimental and atomic simulation data. Furthermore, the model is used to elucidate the effect of nanoparticle size, the magnetic field and the stiffness of the elastic medium on the radial breathing-mode frequencies of several nanoparticles. Our results reveal that the effects of the magnetic field and the elastic medium are significant for nanoparticle with small size. |
| |
| Keywords: | Breathing-mode frequency Nanoparticle Magnetic field Nonlocal elasticity |
| 本文献已被 ScienceDirect 等数据库收录! |
|

