| 二维各向异性磁等离子体的无条件稳定ADE-CNAD-FDTD算法 |
| |
| 引用本文: | 李建雄, 庄永佳, 李现国. 二维各向异性磁等离子体的无条件稳定ADE-CNAD-FDTD算法[J]. 强激光与粒子束, 2018, 30: 012001. doi: 10.11884/HPLPB201830.170269 |
| |
| 作者姓名: | 李建雄 庄永佳 李现国 |
| |
| 作者单位: | 1.天津工业大学 电子与信息工程学院,天津 300387;;2.天津市光电检测技术和系统重点实验室,天津 300387 |
| |
| 基金项目: | 国家自然科学基金项目61372011 |
| |
| 摘 要: | 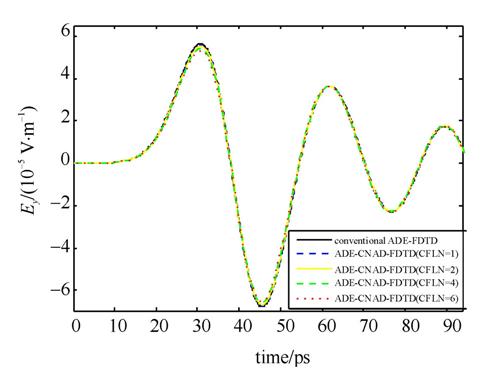
针对二维各向异性磁等离子体提出一种有效的无条件稳定算法,新算法结合了辅助微分方程(ADE)方法与Crank-Nicolson approximate-decoupling(CNAD)时域有限差分算法仿真各向异性磁等离子体介质。传统的ADE-FDTD方法应用在一维各向异性色散介质具有较高的精度和效率,将提出的新算法ADE-CNAD-FDTD应用到二维各向异性磁等离子体介质中不仅解决了电磁波在具有各向异性和频率色散特性介质中传播的仿真难题,而且去除了CFL稳定性条件。该算法在保留了原有的精度情况下大幅度地提高了计算效率并成为无条件稳定的形式。给出一个算例证明该算法的有效性,通过模拟电磁波在磁等离子体中的传播,仿真结果与传统的ADE-FDTD算法对比,证实了该算法的高效率、无条件稳定性和高精度。
|
| 关 键 词: | 辅助微分方程 Crank-Nicolson approximate-decoupling算法 时域有限差分 磁等离子体 |
| 收稿时间: | 2017-06-29 |
| 修稿时间: | 2017-09-22 |
| 本文献已被 CNKI 等数据库收录! |
| 点击此处可从《强激光与粒子束》浏览原始摘要信息 |
|
点击此处可从《强激光与粒子束》下载全文 |
|

